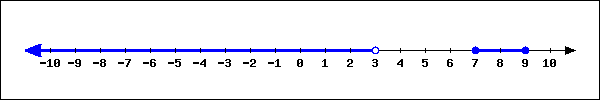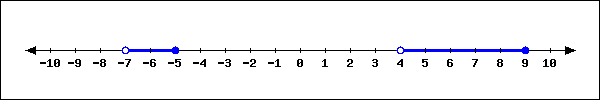Exercises

\(\displaystyle{\begin{aligned}[t] {{\frac{2}{3}}} + {{\frac{3}{4}}} \div {{\frac{5}{4}}} - {{\frac{2}{3}}}\amp ={{\frac{2}{3}}} + {{\frac{3}{4}}} \cdot {{\frac{4}{5}}} - {{\frac{2}{3}}}\\ \amp ={{\frac{2}{3}}}+{{\frac{3}{5}}}-{{\frac{2}{3}}}\\ \amp = \frac{10}{15} + \frac{9}{15} - \frac{10}{15} \\ \amp = \frac{3}{5} \end{aligned} }\)

🔗

Checkpoint 1.6.3.

Divide one fraction into the other: \(\displaystyle{ \frac{7}{6} \div \left(-\frac{5}{4}\right) }\)

🔗

If needed, use an improper fraction in your answer. Don’t use a mixed number.

🔗

Answer.

\(-{\frac{14}{15}}\)

🔗

Solution.

When we do division with fractions, as with

🔗

\(\displaystyle{\frac{7}{6} \div \left(-\frac{5}{4}\right) }\)

🔗

we first change division to multiplication, and at the same time, flip the second fraction. In this case, we have

🔗

\(\displaystyle{\frac{7}{6} \cdot \left(-\frac{4}{5}\right) }\)

🔗

Then we do fraction multiplication as usual. The full process is:

🔗

\(\displaystyle{\begin{aligned}[t] \frac{7}{6} \div \left(-\frac{5}{4}\right) \amp = \frac{7}{6} \cdot \left(-\frac{4}{5}\right) \amp \hbox{We can divide the first denominator and the second numerator by 2.}\\ \amp = \frac{7}{3 }\cdot\left(-\frac{2}{5}\right) \\ \amp = -\frac{14}{15} \end{aligned} }\)

🔗

Checkpoint 1.6.4.

Subtract one fraction from the other: \(\displaystyle{\frac{9}{16} - \frac{15}{16}}\)

🔗

When needed, use an improper fraction in your answer. Don’t use a mixed number.

🔗

Answer.

\(-{\frac{3}{8}}\)

🔗

Solution.

To subtract two fractions with the same denominator, we simply subtract the numerators and keep the denominator unchanged.

🔗

\(\displaystyle{\begin{aligned} \frac{9}{16} - \frac{15}{16} \amp = \frac{9 - 15}{16} \\ \amp = \frac{-6}{16} \amp \hbox{We can reduce this by dividing 2 into both the numerator and the denominator. }\\ \amp = -\frac{3}{8} \\ \end{aligned} }\)

🔗

Checkpoint 1.6.5.

Evaluate this expression:

🔗

\(\displaystyle{ \left({{\frac{7}{4}}}-{{\frac{9}{20}}}\right)-5\left({{\frac{9}{20}}}-{{\frac{7}{4}}}\right)= }\)

🔗

Answer.

\({\frac{39}{5}}\)

🔗

Solution.

You might need to go back to previous units to review how to add fractions.

🔗

\(\begin{aligned} \left({{\frac{7}{4}}}-{{\frac{9}{20}}}\right)-5\left({{\frac{9}{20}}}-{{\frac{7}{4}}}\right) \amp = {{\frac{13}{10}}} -5\left({{\frac{9}{20}}}-{{\frac{7}{4}}}\right) \\ \amp = {{\frac{13}{10}}} -5\left({-{\frac{13}{10}}}\right) \\ \amp = {{\frac{13}{10}}} -\left({-{\frac{13}{2}}}\right) \\ \amp = {{\frac{39}{5}}} \end{aligned}\)

🔗

Checkpoint 1.6.6.

Evaluate this expression:

🔗

\(\displaystyle{ 8-2 [ 3-(6+5\cdot4)]= }\)

🔗

Answer.

\(54\)

🔗

Solution.

The bracket [ ] and parentheses ( ) have the same purpose. We usually use [ ] when ( ) are already used in the problem, so we can tell which pair are which.

🔗

\(\displaystyle{\begin{aligned}[t] \amp \phantom{={}} 8-2 [ 3-(6+5\cdot4)] \\ \amp = 8-2 [ 3-(6+20)] \\ \amp = 8-2 [ 3-26 ] \\ \amp = 8-2 [ -23 ]\\ \amp = 8 + 46 \\ \amp = {54} \end{aligned} }\)

🔗

Checkpoint 1.6.7.

Evaluate this expression:

🔗

\(2^4[2^5-2^3(2^1+2^4)] =\)

🔗

Answer.

\(-1792\)

🔗

Solution.

\begin{equation*} \begin{aligned} \amp \phantom{\mathrel{{}={}}}2^4[2^5-2^3(2^1+2^4)] \\ \amp =2^4[2^5-2^3(2+16)] \\ \amp =2^4[2^5-2^3\cdot18] \\ \amp =2^4[2^5-144] \\ \amp =2^4\cdot[-112] \\ \amp ={-1792} \\ \end{aligned} \end{equation*}

🔗

Checkpoint 1.6.8.

Evaluate this expression:

🔗

\(\displaystyle{ 6[16-3(7+8)] = }\)

🔗

Answer.

\(-174\)

🔗

Solution.

\(\begin{aligned} 6[16-3(7+8)] \amp = 6[16-3\cdot15] \\ \amp = 6[16-45] \\ \amp = 6\cdot[-29] \\ \amp = {-174} \end{aligned}\)

🔗

Checkpoint 1.6.9.

Rewrite the following as a perfect square.

🔗

\(169 s^{16} = \big(\) \(\big)^{2}\)

🔗

Answer.

\(13s^{8}\)

🔗

Checkpoint 1.6.10.

The expression

🔗

\(\frac{(2y^{5})^4}{4y^5}\)

🔗

equals \(cy^e\) where the coefficient \(c\) is , the exponent \(e\) of \(y\) is .

🔗

Answer 1.

\(4\)

🔗

Answer 2.

\(15\)

🔗

Checkpoint 1.6.11.

Find \(x\) if \(\displaystyle{\ \frac{(2.8)^x (2.8)^{-3}}{(2.8)^{-6}}=(2.8)^{4} }\)

🔗

\(x =\)

🔗

Answer.

\(1\)

🔗

Solution.

Use properties of exponents to simplify the left side of the equation\(\displaystyle{\begin{gathered} \frac{(2.8)^x (2.8)^{-3}}{(2.8)^{-6}} = (2.8)^{4} \\ (2.8)^x (2.8)^{-3} (2.8)^{ + 6} = (2.8)^{4} \\ (2.8)^{(x - 3 + 6)} = (2.8)^{4}\ . \end{gathered} }\) The two sides are equal if and only if the exponents are equal, so \(\displaystyle{ x - 3 + 6 = 4 }\text{.}\) Solving for \(x\) one obtains \(\displaystyle{ x = {1} }\text{.}\)

🔗

Checkpoint 1.6.12.

Rewrite the following using a single exponent, and simplify. If

🔗

\begin{equation*} \left(8^{9 x}\right)^{8 + 6 n} = 8^{m} \end{equation*}

🔗

then \(m =\)

🔗

Answer.

\(72x+54xn\)

🔗

Checkpoint 1.6.13.

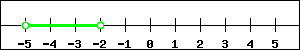
Let \(S=(-3,\infty)\text{,}\) \(T=(-\infty,-1]\text{,}\) and \(W=[-7,-1)\)

🔗

For each intersection or union, choose the correct notation for the resulting interval.

1. \((S \cup T)\)

2. \((S \cup W )\)

3. \((T \cap W)\)

4. \((S \cap T)\)

\(\displaystyle [-7, -1)\)
🔗

🔗
\(\displaystyle (-3, -1]\)
🔗

🔗
\(\displaystyle [-7, \infty)\)
🔗

🔗
\(\displaystyle (-\infty, \infty)\)
🔗

🔗

🔗

Answer 1.

\(\text{D}\)

🔗

Answer 2.

\(\text{C}\)

🔗

Answer 3.

\(\text{A}\)

🔗

Answer 4.

\(\text{B}\)

🔗

Checkpoint 1.6.14.

This exercise is concerned with operations with inequality and interval notations.

🔗

Match the sets and the inequalities by placing the letter of the inequality next to each set described below:

🔗

A
🔗

🔗
B
🔗

🔗
C
🔗

🔗
D
🔗

🔗

1. \(x\) is greater than \(-17\)

🔗

A
🔗

🔗
B
🔗

🔗
C
🔗

🔗
D
🔗

🔗

2. \(x\) is no more than \(8\)

🔗

A
🔗

🔗
B
🔗

🔗
C
🔗

🔗
D
🔗

🔗

3. All \(x\) in the interval \((-17,8]\)

🔗

A
🔗

🔗
B
🔗

🔗
C
🔗

🔗
D
🔗

🔗

4. \(x\) is nonnegative

🔗

A. \(\ \) \(x \leq 8\)

🔗

B. \(\ \) \(-17\lt x\leq 8\)

🔗

C. \(\ \) \(-17\lt x\)

🔗

D. \(\ \) \(x\geq 0\)

🔗

Answer 1.

\(\text{C}\)

🔗

Answer 2.

\(\text{A}\)

🔗

Answer 3.

\(\text{B}\)

🔗

Answer 4.

\(\text{D}\)

🔗

Checkpoint 1.6.15.

Sketch the following sets on a piece of paper and write them in interval notation. Enter the interval in the answer box. You may enter infinity for \(\infty\) and -infinity for \(-\infty\text{.}\) For example, you may write (-infinity, 5] for the interval \((-\infty,5]\text{.}\)

🔗

\({18 \le x \le 21}\) =

🔗

\({22 \lt x \le 27}\) =

🔗

\({-3 \lt x \lt 1}\) =

🔗

\({-4 \le x \lt 1}\) =

🔗

Answer 1.

\(\left[18,21\right]\)

🔗